Sin 2x vs. 2 Sin x: What's the Difference?
Edited by Aimie Carlson || By Janet White || Published on March 2, 2024
sin 2x is a trigonometric function representing the sine of double the angle, whereas 2 sin x doubles the sine of the angle.
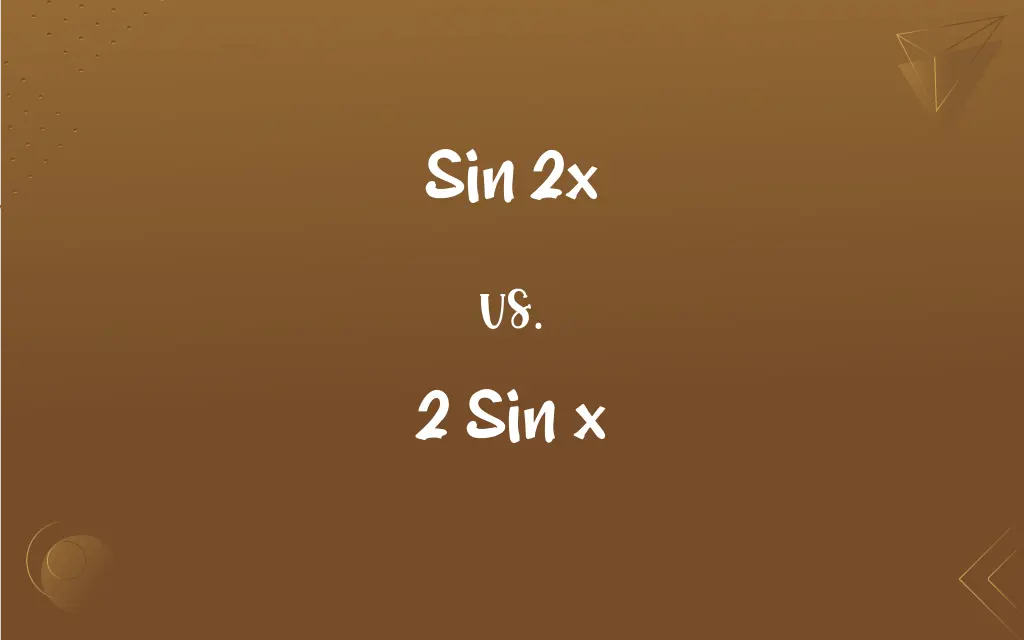
Key Differences
sin 2x and 2 sin x are distinct expressions in trigonometry, each representing different mathematical concepts. sin 2x denotes the sine of twice the angle x, a specific trigonometric function that can be expanded into 2 sin x cos x using the double-angle formula. This function is useful for solving trigonometric equations where angles are doubled. 2 sin x, on the other hand, simply multiplies the sine of angle x by 2. This does not involve any trigonometric identity but rather scales the result of sin x. It is straightforward in its application, directly affecting the amplitude of the sine function, making it twice as large.
The key difference lies in their application and outcome. sin 2x is involved in trigonometric identities and equations that require the manipulation of angle sizes, effectively changing the function's period and pattern. It is fundamental in understanding the relationships between different trigonometric functions.
2 sin x modifies the amplitude of the sine wave without altering the function’s frequency or period. It's a linear transformation of the sine function, used in various physics and engineering contexts where wave amplitude changes are necessary.
Comparing sin 2x and 2 sin x illustrates how trigonometric functions can be manipulated to represent different phenomena. While sin 2x explores the properties of trigonometric identities and their implications on angles, 2 sin x emphasizes the impact of scaling on the sine function's amplitude.
Comparison Chart
Definition
Sine of double the angle
Double the sine of the angle
ADVERTISEMENT
Trigonometric Identity
Uses the double-angle formula: sin 2x = 2 sin x cos x
Not an identity, just a scalar multiplication
Impact on Function
Alters both amplitude and period of the sine wave
Only alters the amplitude, not the period
Usage
In trigonometric identities and solving equations
In scaling the amplitude of sine functions
Mathematical Expression
More complex due to the involvement of cosine
Simpler, as it directly scales the sine value
Sin 2x and 2 Sin x Definitions
Sin 2x
Sin 2x is the sine of twice the angle.
For x = π/4, sin 2x equals 1.
ADVERTISEMENT
2 Sin x
2 sin x scales the amplitude of the sine function.
The amplitude of a wave described by 2 sin x is twice that of sin x.
Sin 2x
Sin 2x transforms the sine function to account for angle doubling.
The graph of sin 2x shows a wave with half the period of sin x.
2 Sin x
2 sin x maintains the period while altering the function's magnitude.
A pendulum's maximum angle might be modeled by 2 sin x to show increased swing.
Sin 2x
Sin 2x applies in scenarios where angle manipulation is key.
In physics, sin 2x helps model oscillations with doubled frequencies.
2 Sin x
2 sin x affects the sine wave without changing its frequency.
Light intensity variations might be modeled as 2 sin x to indicate brighter light.
Sin 2x
Sin 2x represents a double-angle trigonometric function.
Sin 2x can simplify equations involving trigonometric identities.
2 Sin x
2 sin x doubles the value of the sine of angle x.
For x = π/6, 2 sin x equals 1.
Sin 2x
Sin 2x is used in trigonometry to explore relationships between angles.
Sin 2x is integral in proving trigonometric identities.
2 Sin x
2 sin x is a linear modification of the sine function.
In sound engineering, 2 sin x could represent a louder sound wave.
FAQs
What does sin 2x represent?
Sin 2x represents the sine of double the angle, used in trigonometric identities.
Can sin 2x be simplified?
Yes, sin 2x can be simplified to 2 sin x cos x using the double-angle formula.
How does sin 2x affect the sine wave's period?
Sin 2x halves the period of the sine wave, leading to more frequent oscillations.
Is 2 sin x a trigonometric identity?
No, 2 sin x is not an identity but a scaled version of the sine function.
Does 2 sin x change the wave's frequency?
No, 2 sin x does not change the frequency, only the amplitude.
What distinguishes 2 sin x in terms of transformation?
It linearly scales the sine function's output, enhancing its magnitude.
What is the practical use of 2 sin x?
2 sin x is used to model phenomena where the amplitude of a wave needs to be doubled.
How is sin 2x derived?
Sin 2x is derived from the double-angle formula in trigonometry.
How does altering x in 2 sin x affect the wave?
Altering x in 2 sin x affects the phase of the wave, not its amplitude or period.
Why use sin 2x in equations?
Sin 2x is useful for solving trigonometric equations involving angle relationships.
In what scenarios is sin 2x applied?
Sin 2x is applied in mathematical and physical scenarios requiring angle doubling.
Can sin 2x be used to model physical phenomena?
Yes, sin 2x is used in physics to model phenomena with doubled angles or frequencies.
How does 2 sin x differ from sin x?
2 sin x doubles the amplitude of the sine of angle x, without altering the period.
Is sin 2x equivalent to 2 sin x?
Not directly; sin 2x involves a trigonometric identity, while 2 sin x is a scaling factor.
Why is 2 sin x significant in wave theory?
It's significant for adjusting the amplitude, relevant in acoustics and optics.
How does sin 2x influence trigonometric proofs?
It's crucial for demonstrating relationships and identities within trigonometry.
What happens to the sine wave's amplitude with 2 sin x?
The amplitude is doubled, making the wave's peaks and troughs more pronounced.
Can sin 2x be applied in engineering?
Yes, it's applied in signal processing and mechanical oscillations for frequency analysis.
What role does 2 sin x play in mathematical modeling?
It models situations where the intensity or magnitude of a sine-based phenomenon is increased.
What is the impact of 2 sin x on wave properties?
It increases the wave's amplitude without affecting its frequency or period.
About Author
Written by
Janet WhiteJanet White has been an esteemed writer and blogger for Difference Wiki. Holding a Master's degree in Science and Medical Journalism from the prestigious Boston University, she has consistently demonstrated her expertise and passion for her field. When she's not immersed in her work, Janet relishes her time exercising, delving into a good book, and cherishing moments with friends and family.
Edited by
Aimie CarlsonAimie Carlson, holding a master's degree in English literature, is a fervent English language enthusiast. She lends her writing talents to Difference Wiki, a prominent website that specializes in comparisons, offering readers insightful analyses that both captivate and inform.
































































